Conduction-Convection and Radiation from Pipes
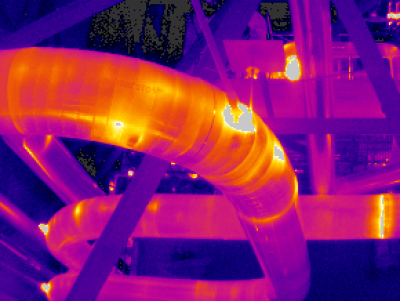
Description
Calculation Reference
Heat Loss from Pipes
Heat Transfer
Fliuid Flow in Pipes
Conduction, convection, and radiation are three different mechanisms of heat transfer. When it comes to heat loss from pipes, these mechanisms play important roles.
- Conduction: Conduction is the transfer of heat through direct contact between objects or substances. In the context of pipes, conduction occurs when heat is conducted from the pipe's hotter surface to its cooler surroundings. The rate of heat transfer by conduction depends on factors such as the temperature difference between the pipe and its surroundings, the thermal conductivity of the pipe material, and the thickness of the pipe wall. Materials with higher thermal conductivity, such as metals, facilitate faster heat transfer through conduction.
To minimize heat loss through conduction from pipes, insulation is often used. Insulating materials with low thermal conductivity, such as foam, fiberglass, or mineral wool, are wrapped around the pipes. This insulation layer helps to reduce the temperature gradient between the pipe and its surroundings, thus reducing heat transfer by conduction.
- Convection: Convection is the transfer of heat through the movement of fluids (liquids or gases). In the case of pipes, convection heat loss can occur when there is a temperature difference between the pipe's surface and the surrounding fluid (e.g., air or water). When the fluid comes into contact with the pipe's surface, it absorbs heat through conduction and then carries it away as it flows. This heat transfer mechanism is known as convective heat transfer.
The rate of heat loss through convection depends on various factors, including the temperature difference, fluid velocity, surface area of the pipe, and properties of the fluid (e.g., viscosity). Increasing the fluid velocity or using a more efficient heat transfer fluid can enhance heat dissipation by convection.
To minimize convective heat loss from pipes, insulation can still play a role. Insulating materials can help reduce heat exchange between the pipe surface and the surrounding fluid, thus reducing convective heat transfer.
- Radiation: Radiation is the transfer of heat through electromagnetic waves. All objects with a temperature above absolute zero emit thermal radiation. In the case of pipes, they can both absorb and emit thermal radiation. The amount of radiation heat loss depends on the temperature of the pipe's surface, the emissivity of the pipe material, and the temperature of the surrounding objects or surfaces.
To minimize heat loss through radiation from pipes, using materials with low emissivity can be beneficial. Reflective coatings or insulation materials with low emissivity properties can reduce the amount of thermal radiation emitted from the pipe's surface. Additionally, maintaining a lower temperature difference between the pipe and its surroundings can help reduce radiation heat loss.
In practice, a combination of insulation techniques, including thermal insulation to minimize conduction and convective heat loss, as well as strategies to reduce radiation heat loss, is often employed to optimize heat conservation and energy efficiency in pipe systems.
Several formulas can be used to quantify heat loss through conduction, convection, and radiation. Here are the commonly used equations for each type of heat transfer:
- Conduction:
The rate of heat transfer through conduction can be calculated using Fourier's Law of Heat Conduction:
Q = (k * A * ΔT) / L
Where: Q is the rate of heat transfer (in watts), k is the thermal conductivity of the material (in watts per meter-kelvin), A is the cross-sectional area of the pipe (in square meters), ΔT is the temperature difference between the pipe and its surroundings (in kelvin), and L is the thickness of the pipe wall (in meters).
- Convection:
The rate of heat transfer through convection can be determined using the convective heat transfer equation:
Q = h * A * ΔT
Where: Q is the rate of heat transfer (in watts), h is the convective heat transfer coefficient (in watts per square meter-kelvin), A is the surface area of the pipe (in square meters), and ΔT is the temperature difference between the pipe surface and the surrounding fluid (in kelvin).
Note that the convective heat transfer coefficient (h) depends on various factors, including the fluid properties, flow conditions, and surface characteristics. It is typically determined through experimental data or obtained from correlations.
- Radiation:
The rate of heat transfer through radiation can be calculated using the Stefan-Boltzmann Law:
Q = ε * σ * A * (T1^4 - T2^4)
Where: Q is the rate of heat transfer (in watts), ε is the emissivity of the pipe surface (a dimensionless quantity between 0 and 1), σ is the Stefan-Boltzmann constant (5.67 x 10^-8 watts per square meter-kelvin^4), A is the surface area of the pipe (in square meters), T1 is the temperature of the pipe surface (in kelvin), and T2 is the temperature of the surrounding objects or surfaces (in kelvin).
Note that the Stefan-Boltzmann Law assumes that the pipe is a perfect blackbody radiator. If the pipe's emissivity is known, it can be used directly. Otherwise, the effective emissivity should be considered, taking into account the pipe's material and surface properties.
These equations provide a basis for calculating heat loss through conduction, convection, and radiation, allowing engineers and designers to optimize insulation and other measures to minimize energy losses in pipe systems.
Calculation Preview
Full download access to any calculation is available to users with a paid or awarded subscription (XLC Pro).
Subscriptions are free to contributors to the site, alternatively they can be purchased.
Click here for information on subscriptions.




