Area Moments of Inertia.xls

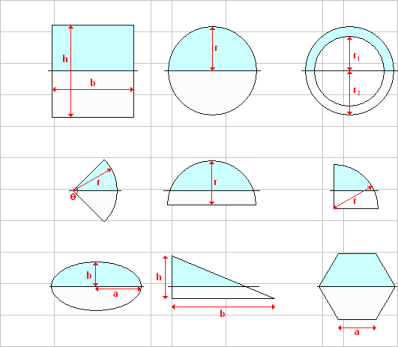
Area Moments of Inertia for some standard shapes

Calculation Reference

The area moment of inertia, also known as the second moment of area, is a property of a shape that measures its resistance to bending and deflection. Here are the area moments of inertia for some standard shapes:

1. Rectangle:

Ix = (b * h^3) / 12 Iy = (h * b^3) / 12

Where: Ix = moment of inertia about the x-axis Iy = moment of inertia about the y-axis b = width of the rectangle h = height of the rectangle

2. Circle:

I = (pi * d^4) / 64 = (pi * r^4) / 4

Where: I = moment of inertia about the central axis d = diameter of the circle r = radius of the circle

3. Hollow Circle (Circular Ring):

I = (pi * (d_o^4 - d_i^4)) / 64 = (pi * (r_o^4 - r_i^4)) / 4

Where: I = moment of inertia about the central axis d_o = outer diameter of the circular ring d_i = inner diameter of the circular ring r_o = outer radius of the circular ring r_i = inner radius of the circular ring

4. I-Beam:

Ix = (1/12) * (b_f * h_f^3 + b_w * h_w^3)

Where: Ix = moment of inertia about the x-axis b_f = width of the flanges h_f = height of the flanges b_w = width of the web h_w = height of the web

5. Triangle:

Ix = (b * h^3) / 36 Iy = (h * b^3) / 48

Where: Ix = moment of inertia about the x-axis Iy = moment of inertia about the y-axis b = base of the triangle h = height of the triangle

These formulas provide the area moments of inertia for various standard shapes, which are essential for calculating stresses, deflections, and other mechanical properties in structural and mechanical engineering applications.