Buoyancy and Drag
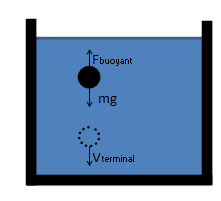
Description
Purpose of calculation:
Buoyancy and drag
Calculation Reference
http://hyperphysics.phy-astr.gsu.edu/hbase/lindrg.html
Calculation Validation
Reproduction of a sample calculation from a published calculation reference.
Calculation Reference
Fluid Mechanics
Viscosity
Hydraulics
Buoyancy and drag are important concepts in fluid mechanics, as they describe how fluid forces act on objects submerged or moving through a fluid medium. Fluid mechanics deals with the behavior of fluids (liquids and gases) at rest or in motion, and understanding these concepts is crucial in many engineering applications, including hydraulics and the design of structures or vehicles in contact with fluids.
- Buoyancy: Buoyancy is the upward force exerted by a fluid on an object submerged in it or floating on its surface. This force is a result of the pressure difference acting on the object's surfaces caused by the fluid's weight. The concept of buoyancy is based on Archimedes' principle, which states that the buoyant force acting on an object submerged in a fluid is equal to the weight of the fluid displaced by the object.
Mathematically, the buoyant force (F_b) can be expressed as:
F_b = ρ_f * V_d * g
where: ρ_f = density of the fluid (kg/m³ or lb/ft³) V_d = volume of the fluid displaced by the object (m³ or ft³) g = acceleration due to gravity (approximately 9.81 m/s² or 32.2 ft/s²)
If the buoyant force equals the weight of the object, the object will float. If the buoyant force is less than the weight of the object, it will sink. If the buoyant force is greater than the weight of the object, it will rise in the fluid.
- Drag: Drag is the resistive force experienced by an object moving through a fluid or when a fluid flows around a stationary object. Drag opposes the object's motion and is influenced by factors such as fluid viscosity, object shape, and relative velocity between the object and the fluid. There are two primary types of drag: viscous (or skin) drag and pressure (or form) drag.
Viscous drag occurs due to the friction between the fluid and the object's surface, while pressure drag is a result of the pressure difference between the front and rear surfaces of the object. The total drag force (F_d) can be expressed as:
F_d = 0.5 * C_d * ρ_f * A * V²
where: C_d = drag coefficient (dimensionless, depends on the object's shape and flow conditions) ρ_f = density of the fluid (kg/m³ or lb/ft³) A = reference area of the object (m² or ft², typically its cross-sectional area) V = relative velocity between the object and the fluid (m/s or ft/s)
Understanding buoyancy and drag is essential for various applications, including the design of ships, submarines, and underwater structures (buoyancy), as well as vehicles, aircraft, and wind turbines (drag).
In fluid mechanics, viscosity plays a crucial role in the analysis of fluid flow. Viscosity is the measure of a fluid's resistance to deformation or flow due to internal friction between its particles. Fluids with high viscosity, like honey, flow more slowly and generate more viscous drag than fluids with low viscosity, like water.
Hydraulics is a branch of fluid mechanics that deals with the behavior of incompressible fluids (usually liquids) in systems such as pipes, pumps, and dams. Buoyancy, drag, and viscosity are important concepts to consider when designing hydraulic systems or analyzing fluid flow within them.
Calculation Preview
Full download access to any calculation is available to users with a paid or awarded subscription (XLC Pro).
Subscriptions are free to contributors to the site, alternatively they can be purchased.
Click here for information on subscriptions.


