Constant Acceleration Motion Calculations.xls

Excel encoded the HyperPhysics Motion page following the conventions and advisory notes set out on www.excelcalcs.com/earn-your-subscription/.

Calculation Reference
Equations of Motion

Kinematics

Constant acceleration motion, also known as uniformly accelerated motion, is a type of motion where an object's velocity changes at a constant rate over time. In other words, the object's acceleration remains constant throughout the motion. This type of motion is often seen in physics problems and real-world scenarios, such as a car accelerating from rest or a freely falling object under the influence of gravity.

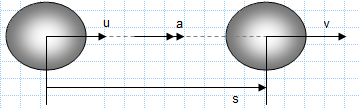
There are four main kinematic equations that describe constant acceleration motion:

1. v = u + at
2. s = ut + 0.5at^2
3. v^2 = u^2 + 2as
4. s = 0.5(u + v)t

In these equations:

• 'v' represents the final velocity of the object
• 'u' represents the initial velocity of the object
• 'a' represents the constant acceleration
• 't' represents the time elapsed
• 's' represents the displacement (change in position) of the object

Here's a brief explanation of each equation:

1. v = u + at: This equation relates the initial and final velocities of the object, the constant acceleration, and the time elapsed. It shows that the final velocity is equal to the initial velocity plus the product of acceleration and time.

2. s = ut + 0.5at^2: This equation relates the displacement of the object to its initial velocity, constant acceleration, and time elapsed. It shows that the displacement is equal to the product of the initial velocity and time, plus half of the product of acceleration and the square of time.

3. v^2 = u^2 + 2as: This equation relates the initial and final velocities, constant acceleration, and displacement of the object. It shows that the square of the final velocity is equal to the square of the initial velocity plus twice the product of acceleration and displacement.

4. s = 0.5(u + v)t: This equation relates the displacement, initial and final velocities, and time elapsed for an object in constant acceleration motion. It shows that the displacement is equal to half the sum of the initial and final velocities multiplied by the time elapsed.

These equations are useful for solving various problems related to constant acceleration motion, such as calculating the time it takes for an object to reach a certain velocity, the distance it travels during that time, or the acceleration needed to achieve a specific change in velocity.